\begin{equation}
f(-x) = f(x)
\end{equation}を満たす関数を偶関数、
\begin{equation}
f(-x) = -f(x)
\end{equation}を満たす関数を奇関数といいます。
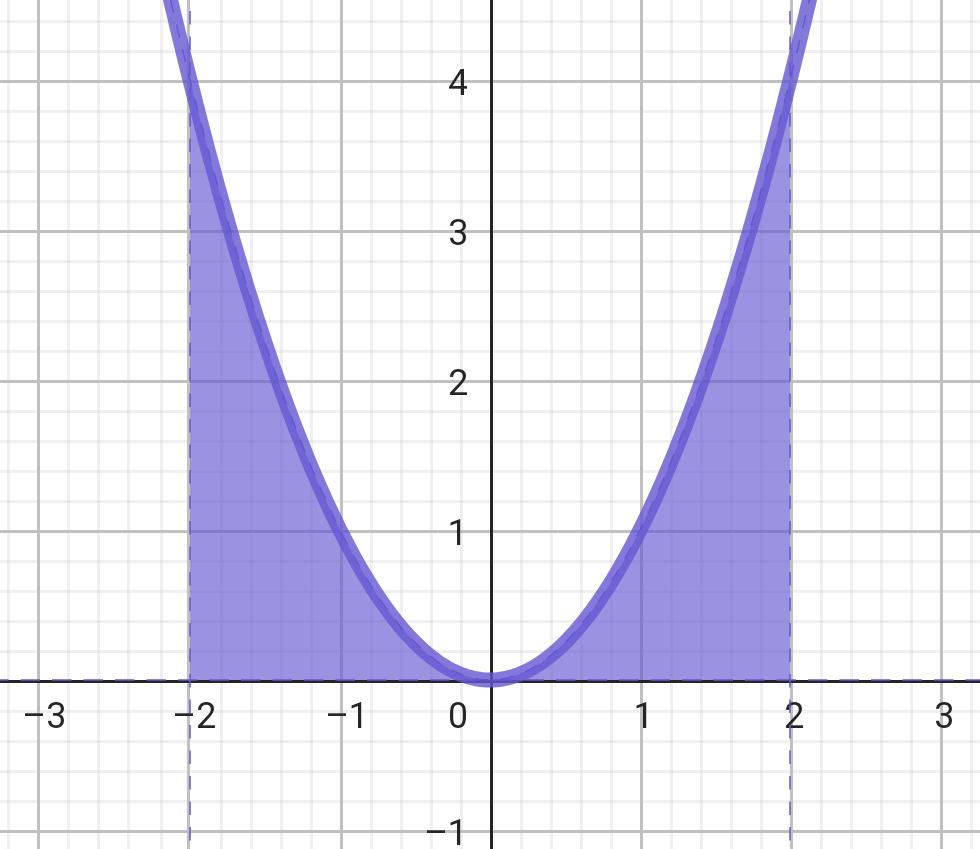
また、が
偶関数のとき
\begin{equation}
\int_{-a}^a f(x) \, dx = 2\int_0^a f(x) \, dx
\end{equation}
奇関数のとき
\begin{equation}
\int_{-a}^a f(x) \, dx = 0
\end{equation}が成り立ちます。
\begin{equation}
\int_{-a}^a f(x) \, dx = \int_{-a}^0 f(x) \, dx +\int_0^a f(x) \, dx
\end{equation}の右辺第1項は、を
を置き換えます。
\begin{eqnarray}
\int_{-a}^0 f(x) \, dx &=& \int_a^0 f(-x) \, d(-x) \\
&=& -\int_a^0 f(-x) \, dx \\
&=& \int_0^a f(-x) \, dx
\end{eqnarray}となります。
偶関数の場合、
\begin{equation}
\int_{-a}^0 f(x) \, dx = \int_0^a f(x) \, dx
\end{equation}なので
\begin{equation}
\int_{-a}^a f(x) \, dx = 2\int_0^a f(x) \, dx
\end{equation}となります。
奇関数の場合、
\begin{equation}
\int_{-a}^0 f(x) \, dx = -\int_0^a f(x) \, dx
\end{equation}なので、
\begin{equation}
\int_{-a}^a f(x) \, dx = 0
\end{equation}となります。
